Find a Member of the Family That Is a Solution With Initial Conditions Y(0) = 1 and Y'(0) = 2.
8.ane: Basics of Differential Equations
- Page ID
- 10782
Learning Objectives
- Identify the society of a differential equation.
- Explain what is meant by a solution to a differential equation.
- Distinguish between the general solution and a item solution of a differential equation.
- Identify an initial-value problem.
- Place whether a given function is a solution to a differential equation or an initial-value problem.
Calculus is the mathematics of alter, and rates of modify are expressed by derivatives. Thus, 1 of the almost common ways to utilize calculus is to set up an equation containing an unknown part \(y=f(x)\) and its derivative, known as a differential equation. Solving such equations oft provides data about how quantities modify and frequently provides insight into how and why the changes occur.
Techniques for solving differential equations can have many different forms, including direct solution, use of graphs, or computer calculations. We innovate the master ideas in this chapter and describe them in a petty more detail later in the course. In this section we study what differential equations are, how to verify their solutions, some methods that are used for solving them, and some examples of common and useful equations.
Full general Differential Equations
Consider the equation \(y′=3x^2,\) which is an example of a differential equation considering information technology includes a derivative. At that place is a relationship between the variables \(ten\) and \(y:y\) is an unknown role of \(x\). Furthermore, the left-hand side of the equation is the derivative of \(y\). Therefore we tin can interpret this equation as follows: Outset with some function \(y=f(x)\) and take its derivative. The answer must be equal to \(3x^ii\). What part has a derivative that is equal to \(3x^ii\)? 1 such function is \(y=10^three\), and so this function is considered a solution to a differential equation.
Definition: differential equation
A differential equation is an equation involving an unknown function \(y=f(10)\) and one or more of its derivatives. A solution to a differential equation is a role \(y=f(x)\) that satisfies the differential equation when \(f\) and its derivatives are substituted into the equation.
Go to this website to explore more on this topic.
Some examples of differential equations and their solutions announced in Table \(\PageIndex{ane}\).
| Equation | Solution |
|---|---|
| \(y'=2x\) | \(y=ten^2\) |
| \(y'+3y=6x+11\) | \(y=e^{−3x}+2x+3\) |
| \(y''−3y'+2y=24e^{−2x}\) | \(y=3e^x−4e^{2x}+2e^{−2x}\) |
Notation that a solution to a differential equation is not necessarily unique, primarily because the derivative of a constant is goose egg. For case, \(y=ten^2+iv\) is besides a solution to the first differential equation in Table \(\PageIndex{1}\). We will return to this idea a little fleck later in this section. For now, let'southward focus on what it ways for a function to be a solution to a differential equation.
Example \(\PageIndex{1}\): Verifying Solutions of Differential Equations
Verify that the function \(y=e^{−3x}+2x+iii\) is a solution to the differential equation \(y′+3y=6x+eleven\).
Solution
To verify the solution, we first calculate \(y′\) using the chain dominion for derivatives. This gives \(y′=−3e^{−3x}+two\). Side by side we substitute \(y\) and \(y′\) into the left-hand side of the differential equation:
\((−3e^{−2x}+2)+3(eastward^{−2x}+2x+3).\)
The resulting expression tin be simplified by outset distributing to eliminate the parentheses, giving
\(−3e^{−2x}+2+3e^{−2x}+6x+9.\)
Combining like terms leads to the expression \(6x+eleven\), which is equal to the right-hand side of the differential equation. This consequence verifies that \(y=e^{−3x}+2x+three\) is a solution of the differential equation.
Practice \(\PageIndex{1}\)
Verify that \(y=2e^{3x}−2x−two\) is a solution to the differential equation \(y′−3y=6x+4.\)
- Hint
-
First calculate \(y′\) then substitute both \(y′\) and \(y\) into the left-mitt side.
It is convenient to define characteristics of differential equations that make information technology easier to talk about them and categorize them. The most basic characteristic of a differential equation is its gild.
Definition: guild of a differential equation
The order of a differential equation is the highest society of whatever derivative of the unknown part that appears in the equation.
Example \(\PageIndex{2}\): Identifying the Club of a Differential Equation
The highest derivative in the equation is \(y′\),
What is the social club of each of the following differential equations?
- \(y′−4y=ten^two−3x+4\)
- \(ten^2y'''−3xy''+xy′−3y=\sin 10\)
- \(\frac{iv}{x}y^{(4)}−\frac{6}{x^2}y''+\frac{12}{10^four}y=10^three−3x^2+4x−12\)
Solution
- The highest derivative in the equation is \(y′\),and then the social club is \(1\).
- The highest derivative in the equation is \(y'''\), and so the order is \(iii\).
- The highest derivative in the equation is \(y^{(4)}\), so the order is \(4\).
Do \(\PageIndex{ii}\)
What is the club of the following differential equation?
\((x^4−3x)y^{(5)}−(3x^2+1)y′+3y=\sin x\cos x\)
- Hint
-
What is the highest derivative in the equation?
- Answer
-
\(5\)
Full general and Detail Solutions
We already noted that the differential equation \(y′=2x\) has at least two solutions: \(y=10^ii\) and \(y=ten^two+4\). The just difference between these two solutions is the terminal term, which is a abiding. What if the last term is a different constant? Will this expression still be a solution to the differential equation? In fact, any function of the form \(y=x^2+C\), where \(C\) represents any constant, is a solution every bit well. The reason is that the derivative of \(x^two+C\) is \(2x\), regardless of the value of \(C\). Information technology can be shown that any solution of this differential equation must be of the form \(y=x^two+C\). This is an example of a general solution to a differential equation. A graph of some of these solutions is given in Figure \(\PageIndex{1}\). (Note: in this graph nosotros used even integer values for C ranging between \(−iv\) and \(4\). In fact, at that place is no restriction on the value of \(C\); it can exist an integer or not.)
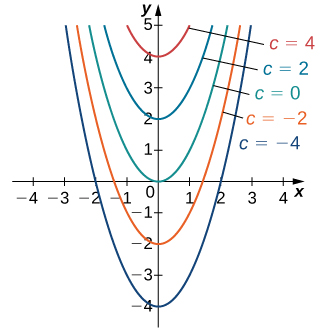
In this example, nosotros are gratuitous to cull whatever solution nosotros wish; for case, \(y=ten^two−3\) is a fellow member of the family of solutions to this differential equation. This is called a particular solution to the differential equation. A particular solution tin often be uniquely identified if we are given additional information about the trouble.
Example \(\PageIndex{3}\): Finding a Particular Solution
Discover the particular solution to the differential equation \(y′=2x\) passing through the signal \((2,7)\).
Solution
Any function of the form \(y=10^2+C\) is a solution to this differential equation. To decide the value of \(C\), we substitute the values \(x=2\) and \(y=7\) into this equation and solve for \(C\):
\[ \begin{align*} y =10^2+C \\[4pt] seven =ii^2+C \\[4pt] =4+C \\[4pt] C =three. \end{align*}\]
Therefore the particular solution passing through the point \((2,7)\) is \(y=10^ii+3\).
Practise \(\PageIndex{3}\)
Discover the particular solution to the differential equation
\[ y′=4x+iii \nonumber\]
passing through the point \((1,seven),\) given that \(y=2x^2+3x+C\) is a full general solution to the differential equation.
- Hint
-
First substitute \(x=1\) and \(y=vii\) into the equation, so solve for \(C\).
- Answer
-
\[ y=2x^2+3x+2 \nonumber\]
Initial-Value Problems
Ordinarily a given differential equation has an space number of solutions, so it is natural to ask which one we want to employ. To choose one solution, more information is needed. Some specific information that can be useful is an initial value, which is an ordered pair that is used to detect a detail solution.
A differential equation together with ane or more initial values is called an initial-value problem. The full general rule is that the number of initial values needed for an initial-value problem is equal to the order of the differential equation. For case, if nosotros take the differential equation \(y′=2x\), then \(y(iii)=7\) is an initial value, and when taken together, these equations grade an initial-value problem. The differential equation \(y''−3y′+2y=4e^x\) is 2nd lodge, then we need ii initial values. With initial-value problems of order greater than one, the same value should be used for the independent variable. An example of initial values for this 2nd-social club equation would be \(y(0)=two\) and \(y′(0)=−ane.\) These 2 initial values together with the differential equation form an initial-value problem. These problems are and then named considering often the independent variable in the unknown function is \(t\), which represents time. Thus, a value of \(t=0\) represents the showtime of the problem.
Example \(\PageIndex{four}\): Verifying a Solution to an Initial-Value Trouble
Verify that the function \(y=2e^{−2t}+e^t\) is a solution to the initial-value problem
\[ y′+2y=3e^t, \quad y(0)=iii.\nonumber\]
Solution
For a part to satisfy an initial-value problem, it must satisfy both the differential equation and the initial condition. To show that \(y\) satisfies the differential equation, we start by computing \(y′\). This gives \(y′=−4e^{−2t}+due east^t\). Next we substitute both \(y\) and \(y′\) into the left-hand side of the differential equation and simplify:
\[ \begin{align*} y′+2y &=(−4e^{−2t}+due east^t)+two(2e^{−2t}+e^t) \\[4pt] &=−4e^{−2t}+eastward^t+4e^{−2t}+2e^t =3e^t. \end{align*}\]
This is equal to the correct-hand side of the differential equation, then \(y=2e^{−2t}+eastward^t\) solves the differential equation. Next nosotros calculate \(y(0)\):
\[ y(0)=2e^{−two(0)}+e^0=2+1=three. \nonumber\]
This consequence verifies the initial value. Therefore the given function satisfies the initial-value problem.
Practise \(\PageIndex{4}\)
Verify that \(y=3e^{2t}+4\sin t\) is a solution to the initial-value trouble
\[ y′−2y=4\cos t−8\sin t,y(0)=3. \nonumber\]
- Hint
-
First verify that \(y\) solves the differential equation. Then cheque the initial value.
In Example \(\PageIndex{4}\), the initial-value trouble consisted of 2 parts. The start function was the differential equation \(y′+2y=3e^x\), and the second office was the initial value \(y(0)=three.\) These two equations together formed the initial-value problem.
The aforementioned is true in full general. An initial-value problem volition consists of 2 parts: the differential equation and the initial condition. The differential equation has a family unit of solutions, and the initial condition determines the value of \(C\). The family unit of solutions to the differential equation in Example \(\PageIndex{four}\) is given past \(y=2e^{−2t}+Ce^t.\) This family unit of solutions is shown in Figure \(\PageIndex{2}\), with the particular solution \(y=2e^{−2t}+e^t\) labeled.
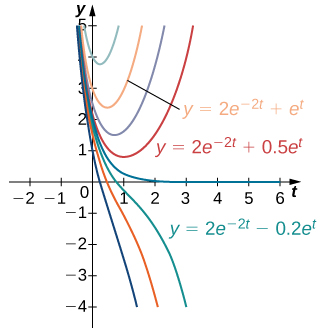
Example \(\PageIndex{v}\): Solving an Initial-value Problem
Solve the post-obit initial-value trouble:
\[ y′=3e^x+x^ii−4,y(0)=5. \nonumber\]
Solution
The first stride in solving this initial-value problem is to find a general family unit of solutions. To do this, we notice an antiderivative of both sides of the differential equation
\[∫y′\,dx=∫(3e^ten+10^2−4)\,dx, \nonumber\]
namely,
\(y+C_1=3e^x+\frac{1}{3}10^3−4x+C_2\).
Nosotros are able to integrate both sides because the y term appears by itself. Find that there are two integration constants: \(C_1\) and \(C_2\). Solving this equation for \(y\) gives
\(y=3e^10+\frac{i}{3}x^3−4x+C_2−C_1.\)
Because \(C_1\) and \(C_2\) are both constants, \(C_2−C_1\) is also a constant. We tin therefore ascertain \(C=C_2−C_1,\) which leads to the equation
\(y=3e^x+\frac{1}{3}x^3−4x+C.\)
Next we make up one's mind the value of \(C\). To practise this, nosotros substitute \(x=0\) and \(y=5\) into this equation and solve for \(C\):
\[ \begin{marshal*} 5 &=3e^0+\frac{1}{3}0^3−4(0)+C \\[4pt] 5 &=3+C \\[4pt] C&=2 \finish{align*}.\]
At present we substitute the value \(C=ii\) into the full general equation. The solution to the initial-value problem is \(y=3e^ten+\frac{1}{3}ten^3−4x+2.\)
Assay
The departure betwixt a full general solution and a detail solution is that a general solution involves a family of functions, either explicitly or implicitly divers, of the independent variable. The initial value or values determine which item solution in the family of solutions satisfies the desired conditions.
Exercise \(\PageIndex{five}\)
Solve the initial-value problem
\[ y′=x^2−4x+iii−6e^10,y(0)=viii. \nonumber \]
- Hint
-
Kickoff take the antiderivative of both sides of the differential equation. Then substitute \(x=0\) and \(y=8\) into the resulting equation and solve for \(C\).
- Respond
-
\(y=\frac{ane}{3}x^iii−2x^2+3x−6e^ten+fourteen\)
In physics and engineering science applications, we often consider the forces acting upon an object, and employ this information to understand the resulting motion that may occur. For instance, if we start with an object at World's surface, the primary forcefulness acting upon that object is gravity. Physicists and engineers tin can apply this information, along with Newton'due south 2nd constabulary of motion (in equation form \(F=ma\), where \(F\) represents force, \(grand\) represents mass, and \(a\) represents acceleration), to derive an equation that tin can be solved.
In Figure \(\PageIndex{three}\) we assume that the only strength acting on a baseball is the forcefulness of gravity. This assumption ignores air resistance. (The force due to air resistance is considered in a after word.) The dispatch due to gravity at Earth'southward surface, thousand, is approximately \(9.8\,\text{yard/s}^ii\). We introduce a frame of reference, where World's surface is at a height of 0 meters. Let \(v(t)\) stand for the velocity of the object in meters per 2nd. If \(v(t)>0\), the ball is rising, and if \(five(t)<0\), the ball is falling (Figure).
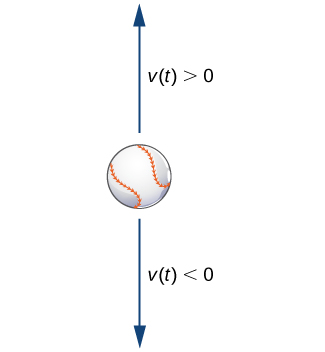
Our goal is to solve for the velocity \(five(t)\) at whatever time \(t\). To do this, we ready an initial-value problem. Suppose the mass of the brawl is \(grand\), where \(thou\) is measured in kilograms. We use Newton's second police, which states that the force interim on an object is equal to its mass times its acceleration \((F=ma)\). Acceleration is the derivative of velocity, then \(a(t)=v′(t)\). Therefore the force acting on the baseball is given by \(F=mv′(t)\). All the same, this force must be equal to the forcefulness of gravity acting on the object, which (again using Newton's second law) is given by \(F_g=−mg\), since this force acts in a downward direction. Therefore nosotros obtain the equation \(F=F_g\), which becomes \(mv′(t)=−mg\). Dividing both sides of the equation past \(1000\) gives the equation
\[ v′(t)=−g.\]
Notice that this differential equation remains the same regardless of the mass of the object.
We now need an initial value. Considering we are solving for velocity, it makes sense in the context of the trouble to assume that nosotros know the initial velocity, or the velocity at time \(t=0.\) This is denoted by \(v(0)=v_0.\)
Example \(\PageIndex{6}\): Velocity of a Moving Baseball
A baseball game is thrown up from a height of \(3\) meters above Globe'southward surface with an initial velocity of \(10\) one thousand/s, and the only force acting on it is gravity. The brawl has a mass of \(0.15\) kg at Earth's surface.
- Find the velocity \(5(t)\) of the basevall at time \(t\).
- What is its velocity after \(2\) seconds?
Solution
a. From the preceding give-and-take, the differential equation that applies in this state of affairs is
\(5′(t)=−g,\)
where \(g=ix.8\, \text{m/s}^ii\). The initial condition is \(v(0)=v_0\), where \(v_0=ten\) chiliad/s. Therefore the initial-value problem is \(v′(t)=−9.8\,\text{thou/southward}^two,\,v(0)=10\) m/south.
The kickoff step in solving this initial-value problem is to take the antiderivative of both sides of the differential equation. This gives
\[\int v′(t)\,dt=∫−9.viii\,dt \nonumber \]
\(v(t)=−9.8t+C.\)
The next pace is to solve for \(C\). To do this, substitute \(t=0\) and \(v(0)=ten\):
\[ \begin{align*} five(t) &=−ix.8t+C \\[4pt] five(0) &=−9.viii(0)+C \\[4pt] 10 &=C. \end{marshal*}\]
Therefore \(C=ten\) and the velocity part is given past \(v(t)=−9.8t+10.\)
b. To find the velocity after \(ii\) seconds, substitute \(t=2\) into \(v(t)\).
\[ \brainstorm{align*} v(t)&=−9.8t+10 \\[4pt] v(2)&=−nine.8(2)+x \\[4pt] v(2) &=−9.6\cease{align*}\]
The units of velocity are meters per second. Since the reply is negative, the object is falling at a speed of \(9.6\) one thousand/due south.
Practise \(\PageIndex{6}\)
Suppose a rock falls from rest from a height of \(100\) meters and the only forcefulness acting on it is gravity. Find an equation for the velocity \(5(t)\) as a function of time, measured in meters per second.
- Hint
-
What is the initial velocity of the rock? Use this with the differential equation in Case \(\PageIndex{half dozen}\) to class an initial-value problem, then solve for \(five(t)\).
- Answer
-
\(v(t)=−ix.8t\)
A natural question to ask after solving this type of trouble is how high the object will be above Earth's surface at a given point in time. Allow \(south(t)\) denote the height higher up Earth's surface of the object, measured in meters. Because velocity is the derivative of position (in this example pinnacle), this assumption gives the equation \(southward′(t)=v(t)\). An initial value is necessary; in this case the initial tiptop of the object works well. Allow the initial height exist given past the equation \(southward(0)=s_0\). Together these assumptions give the initial-value problem
\[ s′(t)=5(t),s(0)=s_0.\]
If the velocity function is known, then it is possible to solve for the position function as well.
Example \(\PageIndex{7}\): Top of a Moving Baseball
A baseball is thrown up from a height of \(3\) meters to a higher place Earth's surface with an initial velocity of \(10m/s\), and the only force acting on it is gravity. The ball has a mass of \(0.15\) kilogram at World'south surface.
- Discover the position \(s(t)\) of the baseball game at fourth dimension \(t\).
- What is its height afterwards \(two\) seconds?
Solution
We already know the velocity function for this problem is \(five(t)=−9.8t+10\). The initial height of the baseball is \(three\) meters, so \(s_0=three\). Therefore the initial-value trouble for this example is
To solve the initial-value trouble, we starting time observe the antiderivatives:
\[∫s′(t)\,dt=∫(−nine.8t+ten)\,dt \nonumber \]
\(s(t)=−4.9t^2+10t+C.\)
Next we substitute \(t=0\) and solve for \(C\):
\(s(t)=−4.9t^ii+10t+C\)
\(s(0)=−4.9(0)^2+10(0)+C\)
\(3=C\).
Therefore the position office is \(s(t)=−four.9t^two+10t+three.\)
b. The acme of the baseball afterward \(2\) sec is given by \(s(2):\)
\(southward(2)=−four.9(2)^2+10(2)+3=−4.9(4)+23=3.four.\)
Therefore the baseball is \(3.4\) meters in a higher place World's surface later \(2\) seconds. It is worth noting that the mass of the ball cancelled out completely in the process of solving the problem.
Fundamental Concepts
- A differential equation is an equation involving a function \(y=f(x)\) and one or more of its derivatives. A solution is a function \(y=f(x)\) that satisfies the differential equation when \(f\) and its derivatives are substituted into the equation.
- The order of a differential equation is the highest gild of any derivative of the unknown function that appears in the equation.
- A differential equation coupled with an initial value is called an initial-value trouble. To solve an initial-value problem, commencement find the general solution to the differential equation, then determine the value of the constant. Initial-value issues have many applications in science and engineering.
Glossary
- differential equation
- an equation involving a function \(y=y(ten)\) and one or more of its derivatives
- general solution (or family of solutions)
- the entire set of solutions to a given differential equation
- initial value(southward)
- a value or ready of values that a solution of a differential equation satisfies for a fixed value of the independent variable
- initial velocity
- the velocity at time \(t=0\)
- initial-value problem
- a differential equation together with an initial value or values
- social club of a differential equation
- the highest lodge of any derivative of the unknown office that appears in the equation
- particular solution
- member of a family of solutions to a differential equation that satisfies a particular initial status
- solution to a differential equation
- a function \(y=f(x)\) that satisfies a given differential equation
Contributors and Attributions
-
Gilbert Strang (MIT) and Edwin "Jed" Herman (Harvey Mudd) with many contributing authors. This content by OpenStax is licensed with a CC-By-SA-NC iv.0 license. Download for gratis at http://cnx.org.
Source: https://math.libretexts.org/Courses/Monroe_Community_College/MTH_211_Calculus_II/Chapter_8:_Introduction_to_Differential_Equations/8.1:_Basics_of_Differential_Equations
0 Response to "Find a Member of the Family That Is a Solution With Initial Conditions Y(0) = 1 and Y'(0) = 2."
Post a Comment